Heating Effect Of Current Class 12 Physics | Notes

Heating effect of current:

When current flows through a conductor, heat develops in it. This effect of current is known as heating effect of current.

When current flows through a conductor, the free electrons of the conductor come in motion and collision takes place between them. Due to the collision moving electrons lose their kinetic energy and some part of their kinetic energy is converted into heat energy.

Joule’s law of heating:

Let us consider a conductor of resistance (R). Let an amount of current (I) is passed through the conductor for time ‘t’. According to Joule’s law of heating, the heat developed in the conductor is

(i) directly proportional to square of magnitude of current flowing through the conductor.

i.e. H $\propto $ I² ………….(i)

(ii) directly proportional to resistance of conductor.

i.e. H $\propto $ R …………..(ii)

(iii) directly proportional to the time for which current is passed through the conductor.

i.e. H $\propto $ t …………..(iii)

On combining (i), (ii) and (iii) we get,

H $\propto $ I²Rt

or, H = k I²Rt

Where ‘k’ is proportionality constant and its value is 1 in S.I.

H = I²Rt

The above result also can be written as

H = $\frac{{{I}^{2}}Rt}{J}$, where ‘J’ is Joule’s equivalent and its value is 4.2 joules/calorie.

Derivation for the expression of heat developed in a wire.

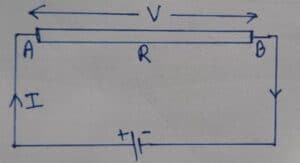
Let us consider a wire AB of resistance R in a circuit as shown in Fig. Let (I) be the steady current passing through it.

In time ‘t’ the total charge ‘q’ passing through B to A is given by

I = $\frac{q}{t}$

or, q = I.t

Let the potential difference between A and B be (V). Then,

V = IR

The work done to transfer the charge (q) from B to A is given by,

W = qV

From equations (i), (ii) and (iii) we get,

W = I t. I R

Or, W = I² R t

It the wire is a passive resistor, all the electrical energy is converted into the heat energy, H.

So, W = H and we have,

H = I²Rt Joule

This is the expression for heat developed in a wire of resistance R.

Experimental verification of Joule’s law of heating:

The experimental setup for verification of Joule’s law of heating is shown in figure above. It consists of a calorimeter with stirrer filled with water about two third of its volume. A heating coil is placed well inside water. The heating coil is connected with a battery through a key, a rheostat (variable resistance) and an ammeter as shown in figure. The variable resistance is used to change the value of current and ammeter is used to measure the value of current.

(i) Verification of first law (i.e. H $\propto $ I²)

Let ‘m’ be the mass of water in the calorimeter of water equivalent ‘W’, specific heat capacity of water is ‘S’.

Now, I₁ amount of current is passed through the heating coil of resistance R for time ‘t’. It is found that the temperature of water and calorimeter increases from $\theta $₁ to $\theta $₂. Now heat developed is

H₁= w($\theta $₂ – $\theta $₁) + ms($\theta $₂ – $\theta $₁)

Let H₂, H₃ and H₄ be the heat developed when different amounts of current I₂, I₃,I₄…………..are passed through the same heating coil for some time, it is found that

$\frac{{{H}_{1}}}{{{I}_{1}}^{2}}$= $\frac{{{H}_{2}}}{{{I}_{2}}^{2}}$= $\frac{{{H}_{3}}}{{{I}_{3}}^{2}}$= $\frac{{{H}_{4}}}{{{I}_{4}}^{2}}$ …

Now, we can write,

$\frac{H}{{{I}^{2}}}$ = constant

It gives H $\propto $ I²

If we plot a graph for different value of H and I², a straight line passing through the origin will be obtained.

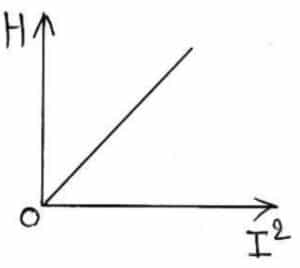
It verifies first law.

(ii) Verification of second law (i.e. H $\propto $ t)

To verify this law, heating coils of different lengths are taken. Let R₁, R₂, R₃, R₄……..be the resistances of different heating coils. If same amount of current is passed through the different heating coils of resistance R_1,R₂, R₃, R₄ for same time the corresponding heat developed is found to be H₁, H₂, H₃, H₄……..respectively. Now, it is found that

$\frac{{{H}_{1}}}{{{R}_{1}}}$= $\frac{{{H}_{2}}}{{{R}_{2}}}$= $\frac{{{H}_{3}}}{{{R}_{3}}}$= $\frac{{{H}_{4}}}{{{R}_{4}}}$ …

Now, we can write,

$\frac{H}{R}$= constant

It gives H $\propto $ R

If we plot a graph for different value of H and R, a straight line passing through the origin will be obtained.

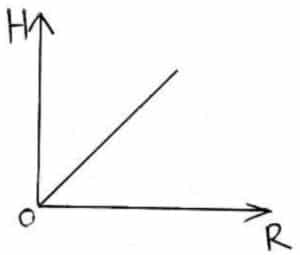
It verifies second law.

(iii) Verification of third law (i.e. H $\propto $ t)

To verify this law, same amount of current is passed through the same heating coil for different time t₁, t₂, t₃, t₄………..the corresponding heat developed are H₁, H₂, H₃, H₄……….respectively. It is found that

$\frac{{{H}_{1}}}{{{t}_{1}}}$= $\frac{{{H}_{2}}}{{{t}_{2}}}$= $\frac{{{H}_{3}}}{{{t}_{3}}}$= $\frac{{{H}_{4}}}{{{t}_{4}}}$………………..

Now, we can write,

$\frac{H}{t}$= constant

It gives H $\propto $ t

If we plot a graph for different value of H and t, a straight line passing through the origin will be obtained.

It verifies third law.

Electric power:

This is defined as the electrical energy per unit time.

Mathematically,

Electric power, P = $\frac{H}{t}$ = $\frac{{{I}^{2}}Rt}{t}$

P = I²R ………(i)

P = I×IR

P = IV ………(ii) Since, V = IR

P = $\frac{V}{R}$ × V Since, I = $\frac{V}{R}$

P = $\frac{{{V}^{2}}}{R}$ …………..(iii)

From (i), (ii) and (iii)

P = I²R = IV = $\frac{{{V}^{2}}}{R}$

One unit or 1 kilowatt-hour of electrical energy:

Electric power is defined as the electrical energy per unit time.

Electric power, P = $\frac{Electric\text{ }energy}{time}$

Electric energy = P×t

If P =1 KW and t = 1 hour

Then,

Electric energy = 1 KW × 1 hr

= 1 KWhr

= 1 unit

Electric energy consumed is said to be one unit if an electric device of 1KW power is used for 1 hour.

Heating Effect of Current Class 12 Physics | Notes